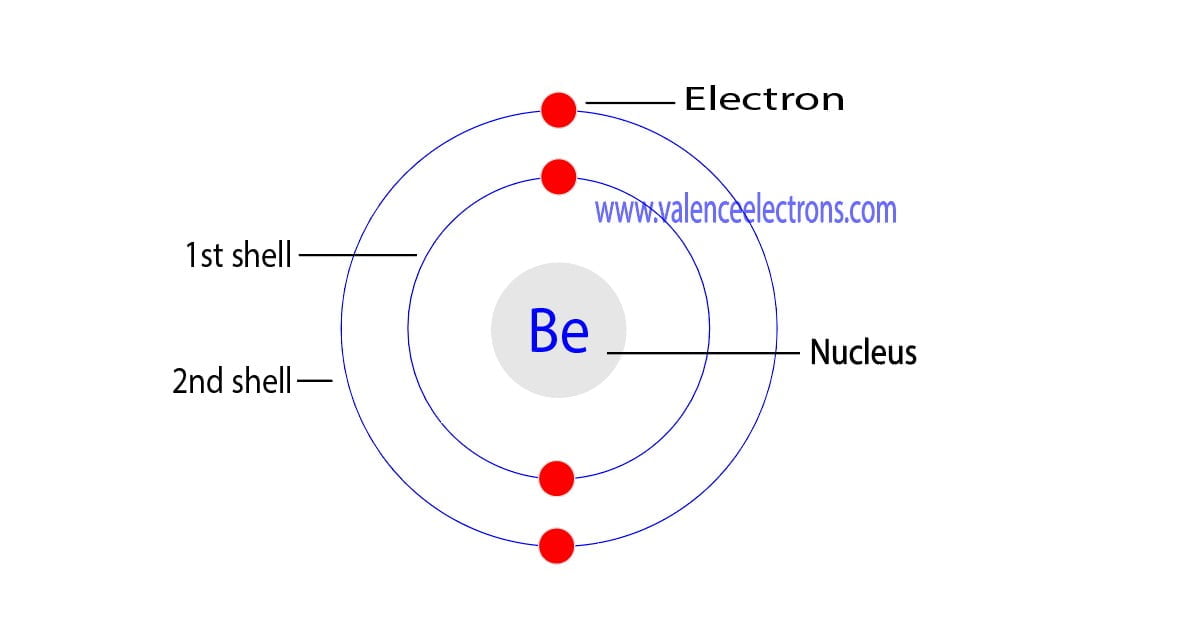
Electron Configuration for Beryllium (Be, Be2+ ion)
Beryllium is the 4th element in the periodic table and its symbol is ‘Be’. In this article, I have discussed in detail how to easily write the complete electron configuration of beryllium.
What is the electron configuration of beryllium?
The total number of electrons in beryllium is four. These electrons are arranged according to specific rules in different orbitals.
The arrangement of electrons in beryllium in specific rules in different orbits and orbitals is called the electron configuration of beryllium.
The electron configuration of beryllium is [He] 2s2, if the electron arrangement is through orbitals. Electron configuration can be done in two ways.
- Electron configuration through orbit (Bohr principle)
- Electron configuration through orbital (Aufbau principle)
Electron configuration through orbitals follows different principles. For example Aufbau principle, Hund’s principle, and Pauli’s exclusion principle.
Electron configuration of Beryllium through orbit
Scientist Niels Bohr was the first to give an idea of the atom’s orbit. He provided a model of the atom in 1913. The complete idea of the orbit is given there.
The electrons of the atom revolve around the nucleus in a certain circular path. These circular paths are called orbits (shell). These orbits are expressed by n. [n = 1,2,3,4 . . . The serial number of the orbit]
K is the name of the first orbit, L is the second, M is the third, and N is the name of the fourth orbit. The electron holding capacity of each orbit is 2n2.
| Shell Number (n) | Shell Name | Electrons Holding Capacity (2n2) |
| 1 | K | 2 |
| 2 | L | 8 |
| 3 | M | 18 |
| 4 | N | 32 |
For example,
- n = 1 for K orbit.
The maximum electron holding capacity in the K orbit is 2n2 = 2 × 12 = 2. - For L orbit, n = 2.
The maximum electron holding capacity in the L orbit is 2n2 = 2 × 22 = 8. - n=3 for M orbit.
The maximum electron holding capacity in the M orbit is 2n2 = 2 × 32 = 18. - n=4 for N orbit.
The maximum electron holding capacity in the N orbit is 2n2 = 2 × 42 = 32.
Therefore, the maximum electron holding capacity in the first shell is two, the second shell is eight and the 3rd shell can have a maximum of eighteen electrons. The atomic number is the number of electrons in that element.
The atomic number of beryllium is 4. That is, the number of electrons in beryllium is 4. Therefore, a beryllium atom will have two electrons in the first shell and two in the 2nd shell.
Therefore, the order of the number of electrons in each shell of the beryllium atom is 2, 2. Electrons can be arranged correctly through orbits from elements 1 to 18.
The electron configuration of an element with an atomic number greater than 18 cannot be properly determined according to the Bohr atomic model. The electron configuration of all the elements can be done through the orbital diagram.
Electron configuration of beryllium through orbital
Atomic energy shells are subdivided into sub-energy levels. These sub-energy levels are also called orbital. The most probable region of electron rotation around the nucleus is called the orbital.
The sub-energy levels depend on the azimuthal quantum number. It is expressed by ‘l’. The value of ‘l’ is from 0 to (n – 1). The sub-energy levels are known as s, p, d, and f.
| Orbit Number | Value of ‘l’ | Number of subshells | Number of orbital | Subshell name | Electrons holding capacity | Electron configuration |
| 1 | 0 | 1 | 1 | 1s | 2 | 1s2 |
| 2 | 0 1 | 2 | 1 3 | 2s 2p | 2 6 | 2s2 2p6 |
| 3 | 0 1 2 | 3 | 1 3 5 | 3s 3p 3d | 2 6 10 | 3s2 3p6 3d10 |
| 4 | 0 1 2 3 | 4 | 1 3 5 7 | 4s 4p 4d 4f | 2 6 10 14 | 4s2 4p6 4d10 4f14 |
For example,
- If n = 1,
(n – 1) = (1–1) = 0
Therefore, the value of ‘l’ is 0. So, the sub-energy level is 1s. - If n = 2,
(n – 1) = (2–1) = 1.
Therefore, the value of ‘l’ is 0, 1. So, the sub-energy levels are 2s, and 2p. - If n = 3,
(n – 1) = (3–1) = 2.
Therefore, the value of ‘l’ is 0, 1, 2. So, the sub-energy levels are 3s, 3p, and 3d. - If n = 4,
(n – 1) = (4–1) = 3
Therefore, the value of ‘l’ is 0, 1, 2, 3. So, the sub-energy levels are 4s, 4p, 4d, and 4f. - If n = 5,
(n – 1) = (n – 5) = 4.
Therefore, l = 0,1,2,3,4. The number of sub-shells will be 5 but 4s, 4p, 4d, and 4f in these four subshells it is possible to arrange the electrons of all the elements of the periodic table.
| Sub-shell name | Name source | Value of ‘l’ | Value of ‘m’ (0 to ± l) | Number of orbital (2l+1) | Electrons holding capacity 2(2l+1) |
| s | Sharp | 0 | 0 | 1 | 2 |
| p | Principal | 1 | −1, 0, +1 | 3 | 6 |
| d | Diffuse | 2 | −2, −1, 0, +1, +2 | 5 | 10 |
| f | Fundamental | 3 | −3, −2, −1, 0, +1, +2, +3 | 7 | 14 |
The orbital number of the s-subshell is one, three in the p-subshell, five in the d-subshell and seven in the f-subshell. Each orbital can have a maximum of two electrons.
The sub-energy level ‘s’ can hold a maximum of two electrons, ‘p’ can hold a maximum of six electrons, ‘d’ can hold a maximum of ten electrons, and ‘f’ can hold a maximum of fourteen electrons.

Aufbau is a German word, which means building up. The main proponents of this principle are scientists Niels Bohr and Pauli. The Aufbau method is to do electron configuration through the sub-energy level.
The Aufbau principle is that the electrons present in the atom will first complete the lowest energy orbital and then gradually continue to complete the higher energy orbital.
The energy of an orbital is calculated from the value of the principal quantum number ‘n’ and the azimuthal quantum number ‘l’. The orbital for which the value of (n + l) is lower is the low energy orbital and the electron will enter that orbital first.
| Orbital | Orbit (n) | Azimuthal quantum number (l) | Orbital energy (n + l) |
| 3d | 3 | 2 | 5 |
| 4s | 4 | 0 | 4 |
Here, the energy of 4s orbital is less than that of 3d. So, the electron will enter the 4s orbital first and enter the 3d orbital when the 4s orbital is full.
The method of entering electrons into orbitals through the Aufbau principle is 1s 2s 2p 3s 3p 4s 3d 4p 5s 4d 5p 6s 4f 5d 6p 7s 5f 6d. The first two electrons of beryllium enter the 1s orbital.
The s-orbital can have a maximum of two electrons. So, the next two electrons enter the 2s orbital. Therefore, the beryllium complete electron configuration will be 1s2 2s2.
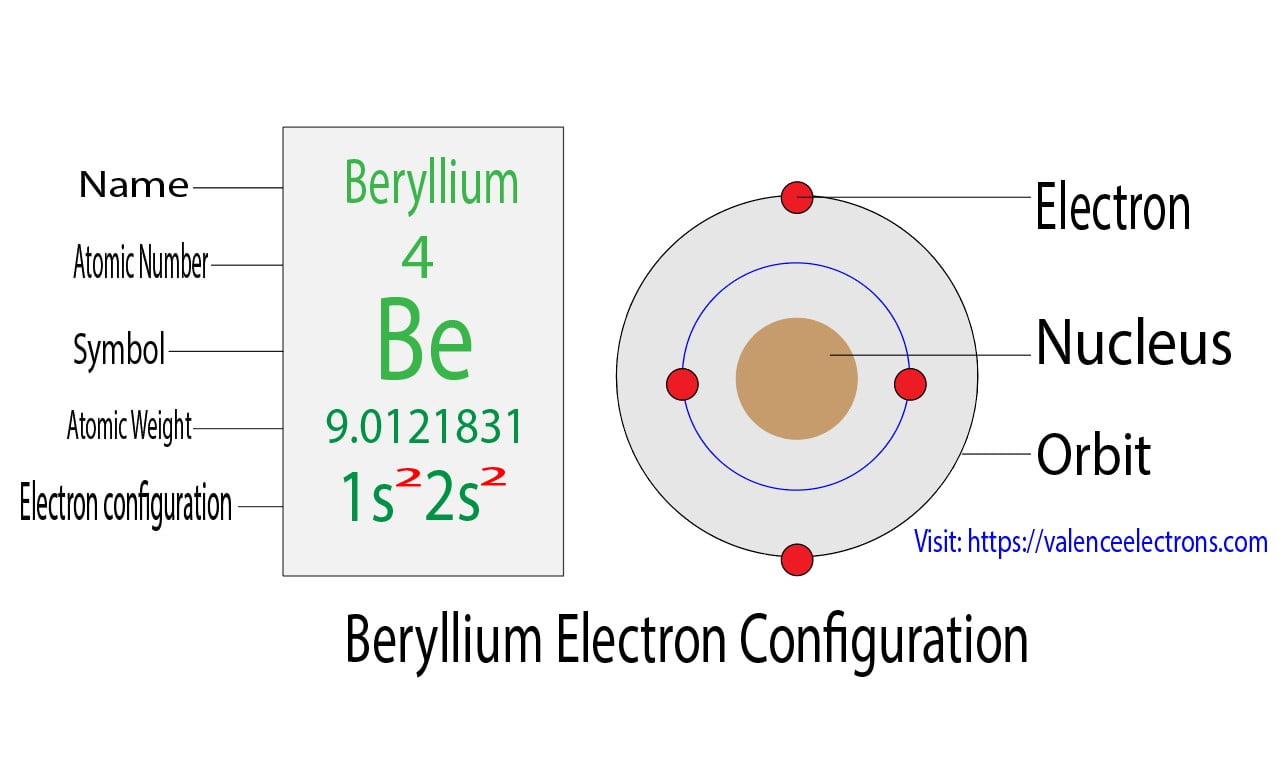
Note: The unabbreviated electron configuration of beryllium is [He] 2s2. When writing an electron configuration, you have to write serially.
Electron configuration of beryllium in the excited state
Atoms can jump from one orbital to another in an excited state. This is called quantum jump. The ground-state electron configuration of beryllium is 1s2 2s2.
When the beryllium atom is excited, then the beryllium atom absorbs energy. As a result, an electron in the 2s orbital jumps to the 2px orbital.

The p-subshell has three orbitals. The orbitals are px, py, and pz. We already know that each orbital can have a maximum of two electrons. Therefore, the electron configuration of beryllium(Be*) in excited state will be 1s2 2s1 2px1.
The valency of an element is determined by electron configuration in the excited state. Here, beryllium has two unpaired electrons. Therefore, the valency of beryllium is 2.
Beryllium ion(Be2+) electron configuration
After the electron configuration, the last shell of the beryllium atom has two electrons. Therefore, the valence electrons of beryllium are two.
The elements that have 1, 2, or 3 electrons in the last shell donate the electrons in the last shell during bond formation. A beryllium atom donates two electrons of the last shell to turn into a beryllium ion(Be2+).
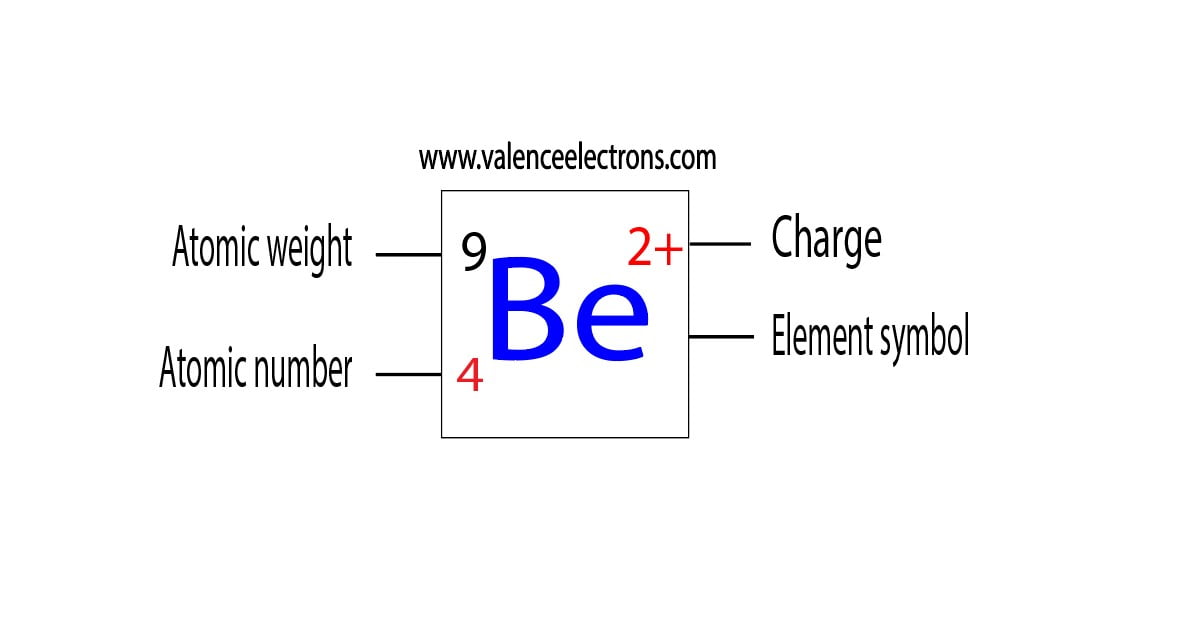
The elements that form bonds by donating electrons are called cation. Beryllium leaves two electrons and turns into a positive ion. Therefore, beryllium is a cation element.
Be – 2e– → Be2+
Here, the electron configuration of beryllium ion(Be2+) is 1s2. This electron configuration shows that the beryllium ion(Be2+) acquired the electron configuration of helium and it achieves a stable electron configuration.
Determine the group and period of beryllium through the electron configuration
The last orbit of an element is the period of that element. The electron configuration of the beryllium atom shows that the last orbit of the beryllium atom is 2. So, the period of beryllium is 2.
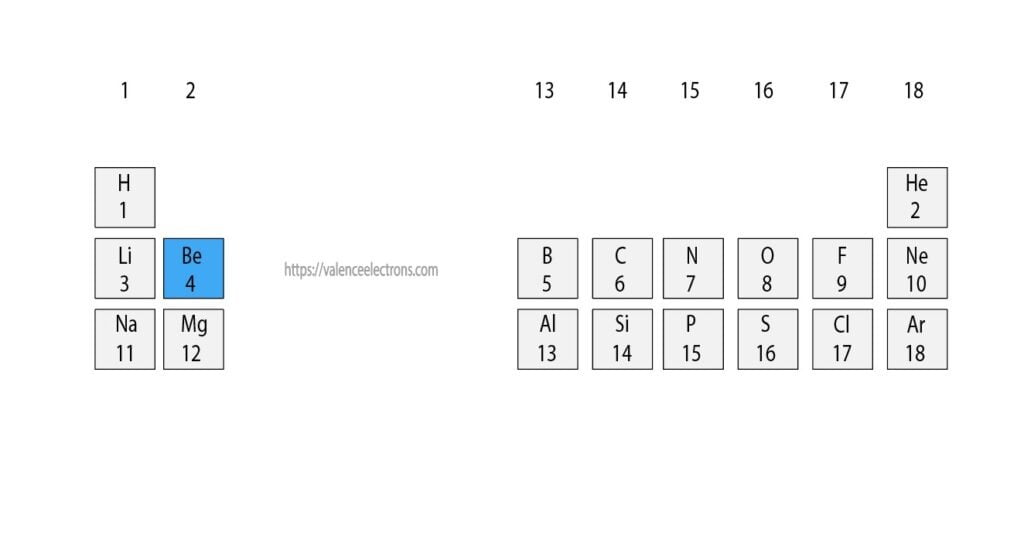
On the other hand, the number of electrons present in the last orbit of an element is the number of groups in that element. Two electrons exist in the last orbit of the beryllium.
That is, the group number of beryllium is 2. Therefore, we can say that the period of the beryllium element is 2 and the group is 2.
Determine the block of beryllium through the electron configuration
If the last electron enters the s-orbital after the electron configuration of the element, then that element is called the s-block element. The electron configuration of beryllium shows that the last electron of beryllium enters the s-orbital. Therefore, beryllium is the s-block element.
The elements in groups-1 and 2 are the s-block elements. And helium is the s-block element. There are 14 s-block elements in the 118 elements of the periodic table.
The elements of group-2 of the periodic table are alkaline soil metals. The first element of group-2 is beryllium. The element of group-2 is beryllium. Therefore, beryllium is an alkaline soil metal.
Ionic bonds of the beryllium atom
The beryllium atom forms ionic bonds by exchanging electrons with the fluorine atom. BeF2 is formed by the exchange of electrons between an atom of beryllium and two atoms of fluorine.
The electron configuration shows that there are two electrons in the last orbit of the beryllium atom. The beryllium atom wants to be more stable by forming one helium atom by eliminating two electrons in the last orbit.
Again, seven electrons exist in the last orbit of the fluorine atom. The fluorine atom wants to be more stable like the neon atom by accepting an electron. The beryllium atom donates two electrons of its last orbit to the fluorine atom. Beryllium fluoride(BeF2) forms compounds through the ionic bond.
Chemical bonding of beryllium
Beryllium oxide
Beryllium reacts with oxygen at high temperatures to produce BeO.
2Be + O2 → 2BeO
However, BeO is less important due to its low solubility.
BeO + H2O → Be(OH)2
BeO reacts with water to produce alkali. However, it destroys the alkalinity of the alkali and the acidity of the acid. BeO reacts with NaOH to form sodium beryllate (Na2BeO2).
BeO + 2NaOH → Na2BeO2 + H2O
That is, BeO is both religious oxides. Beryllium is the s-block element of the 2nd group of the periodic table. Beryllium is an exception compared to other elements in the 2nd group of the phase table. The ion of the beryllium atom has a small size and high ionic potential.
The element has a high polarization capacity of cation and the absence of zero d-orbital at the valence level. For this reason, oxides of other elements in the second group are alkaline but BeO exhibits both properties.
Formation of oxygen compounds
Beryllium is the alkaline soil metal of group-2. It reacts very slowly with oxygen as it is electrically positive. And forms oxide compounds.
2Be + O2 → 2BeO
As the cation size of beryllium increases, the tendency to form peroxide compounds increases.
2BeO + O2 (500 ° C) → 2BeO2
Properties of the beryllium atom
- The atomic number of beryllium is 4. The atomic number of an element is the number of electrons in that element. Therefore, the number of electrons in beryllium is four.
- Beryllium’s standard atomic weight is 9.012183.
- Beryllium is an alkaline soil metal.
- The period of the beryllium element is 2 and the group is 2.
- The number of valency and valence electrons of a beryllium atom is two.
- Beryllium is an s-block element.
- Beryllium easily leaves two electrons in its last orbit and turns into a cation. Therefore, beryllium is a very active metal.
- The beryllium atom forms both ionic and covalent bonds.
- The beryllium atom is relatively small and its ionic energy value is relatively high. For this, its electrons cannot be elevated to high energy levels under the influence of flame energy. For this reason, the beryllium element flame test does not show any particular color.
- Ionization energies of beryllium atoms- 1st: 899.5 kJ/mol, 2nd: 1757.1 kJ/mol, 3rd: 14,848.7 kJ/mol
- The oxidation state of the beryllium atom is 2.
- The melting point of a beryllium atom is 1560 K (1287 °C, 2349 °F). And the boiling point is 2742 K (2469 °C, 4476 °F)
- The atomic radius of a beryllium atom is 112 pm.
- The value of electronegativity of a beryllium atom is 1.57.
- The covalent radius of the beryllium atom is 96±3 pm.
- Beryllium atom van der Waals radius 153 pm.
- 2 electrons exist in the last orbit of beryllium.